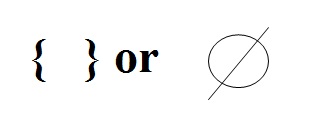Chapter 1
Section 1.1
1) Set definition:
Set is a collection of objects, animals, people or things.
Objects that form a set are called elements.
Notation:
For sets, we use the uppercase letters A, B, C, D, E, ....
For elements, we use the lower case letters a, b, c, d, e, ....
2) To Belong
Definition: We use the pertinence to define whether an element belongs or not
to a given set.
When the element belongs to the set, we use the symbol:

When the element does not belong to the set, we use the symbol:
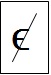
Examples:

3) Equality of Sets
Definition: two sets are said to be equal when they have the same elements.
Notation:
A = B (The set A is equal to B)
4) Empty Set
Definition: is that set that has no element whatsoever.
Notation:
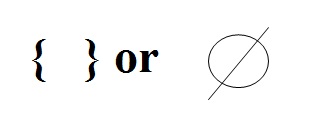
5) Universe Set
Definition: it is the one that has all the elements of all the sets we are studying.
Notation:
U (Universe Set)
6) Subsets
Definition: We have two sets A and B. We say that B is a subset of A, when all elements of B belong to the set A.
Notation:

Main Page
Next Class